The diameter of a circle is 8 miles. What is the circle's circumference? | Use 3.14 for π.
Question
Answer:
Answer:The formula for solving this problem is 2 pi r x C/360, where pi= 3.14 and r is the radius of a circle. C is the degree of the arc.Substituting the numbers into the problem, you get:2(3.14)3 x 180/360= 6(3.14) x 0.5= 9.42 miles.Step-by-step explanation:The length of an arc depends on the radius of a circle and the central angle Θ. We know that for the angle equal to 360 degrees (2π), the arc length is equal to circumference. Hence, as the proportion between angle and arc length is constant, we can say that:L / Θ = C / 2πAs circumference C = 2πr, Decide on the radius of your circle. For example, it can be equal to 15 cm. (You can also input the diameter into the area of a sector calculator instead.) What will be the angle between the ends of the arc? Let's say it is equal to 45 degrees, or π/4. Calculate the arc length according to the formula above: L = r * Θ = 15 * π/4 = 11.78 cm. Calculate the area of a sector: A = r² * Θ / 2 = 15² * π/4 / 2 = 88.36 cm². You can also use the arc length calculator to find the central angle or the radius of the circle. Simply input any two values into the appropriate boxes and watch it conducting all calculations for you.L / Θ = 2πr / 2πL / Θ = r We find out the arc length formula when multiplying this equation by Θ:L = r * ΘHence, the arc length is equal to radius multiplied by the central angle (in radians).
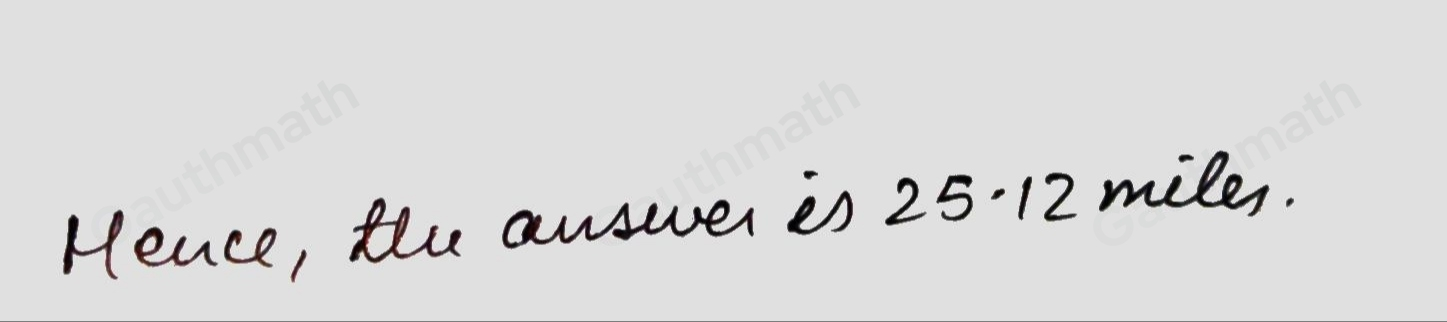
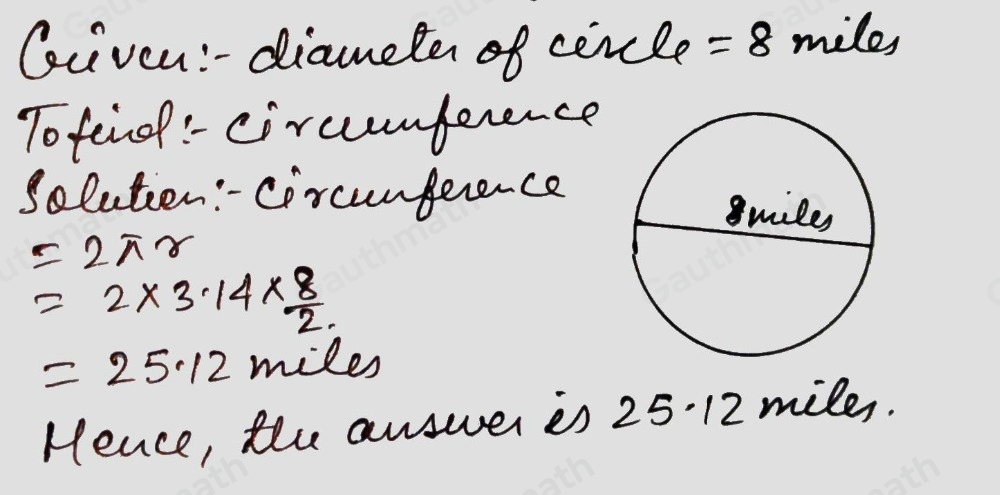
solved
geometry
10 months ago
6711